IBDP Mathematics (AI) : Applications and Interpretations ( HL/SL)
The Mathematics: Application and Interpretation course has been developed to meet the requirements of the new IB Diploma course. These modules emphasize the applied nature of mathematics and is designed for the students who wish to relate how math is related to the world. Students who take this course will be interested in developing their skills in solving practical problems, harnessing technology and exploring mathematical models. This course is suitable for students who may go on to further study in subjects that utilise mathematics in this way such as social sciences, natural sciences, statistics, business, psychology or design.
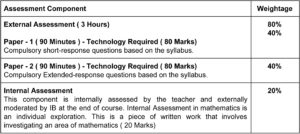
Source: IB Mathematics Analysis and Approaches Guide (AI) 2019
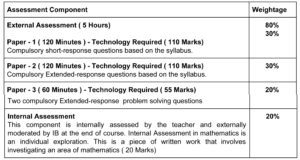
Source: IB Mathematics Analysis and Approaches Guide (AI) 2019
MODULES : View - Register - Learn
In this module, students extensively learn about operations with numbers. Modelling real-life situations with the structure of arithmetic and geometric sequences and series allows for prediction, analysis and interpretation. Students will gain in-depth knowledge of mathematical financial models such as compounded growth and investments. Students benefit from learning deeper insights to do well in the three exam papers.
Prior Learning: Students are expected to be well versed with the concepts of arithmetic and geometric sequences. An understanding of the difference between both the sequences.
Technology: TI-nspire software
Content:
Arithmetic Sequences and series : use of formulae for the nth term and the sum of first n terms of sequence, Sigma Notation and applications.
Analysis, interpretation and prediction where a model is not perfectly arithmetic in real life.
Geometric Sequence and Series: Use of formulae for the nth term and the sum of first n terms of sequence, Sigma Notation and applications.
Financial Applications: Compound Interest and Annual Depreciation.
Duration of the module: 4 Hours (Two sessions of 2 hours each)
Fee: SGD 160/-
Module Code: IBMAIM1
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn the operations on Exponents and logarithms, Approximations and Bounded numbers. Students learn amortization and annuities using technology. The concept of the system of linear equations in three variables and Polynomials are dealt in detail. Students also benefit from learning deeper insights to do well in the three exam papers.
Prior Learning: Students are expected to be well versed with the concepts of financial math: simple and compound interest
Technology: TI-nspire software
Content:
Laws of exponents with integer exponents and logarithms with base 10 and e- Numerical evaluation
Approximating errors, lower and upper bounds, percentage errors and estimation
Amortization and annuities using technology
System of linear equation up to three variables and polynomials
Duration of the module: 4 Hours (Two sessions of 2 hours each)
Fee: SGD 160/-
Module Code: IBMAIM2
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the students learn the concept of sum of infinite geometric sequences and are introduced to the complex numbers, its characteristics and functions. The concept of complex numbers is interpreted in Geometric form. This module helps the students to do well in the paper 2 and paper 3.
Prior Learning: Students are expected to have an understanding of Geometric sequences and series
Technology: TI-nspire software
Content:
Sum of infinite geometric sequences
Introduction to complex numbers: Imaginary, conjugate, modulus and argument.
The complex plane: quadratic solutions, Modulus-argument form, Exponential form
Conversions between cartesian and exponential forms by hand and technology.
Phase shift and Frequencies in the complex plane
Geometric Interpretation of Complex numbers
Duration of the module: 6 Hours (Three sessions of 2 hours each)
Fee: SGD 240/-
Module Code: IBMAIM3
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student will learn the new concept in the IB math course- Matrices and Eigen values. Students will be taught extensively on the role of matrices and its utility in different math components. The concept of eigen values and characteristic polynomials with applications will be discussed. This module helps the students to do well in the paper 2 and paper 3.
Content:
Introduction to Matrices- Operations on matrices, solving equations using matrices
Eigen Values and Eigen Vectors – Characterisation of 2×2 matrices
Diagonalization and applications to powers of 2×2 matrices.
Duration of the module: 4 Hours (2 sessions of two hours each)
Fee: SGD 160/-
Module Code: IBMAIM4
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn to model real-life events using functions. The students get a clear understanding of how to move from different forms of functions. Students also gain a clear insight on function and its inverse and the key features of a graph. This module helps the students to do well in the three exam papers.
Prior Learning: Students are expected to be well versed with forming an equation and solving for the variable and about domain and range.
Technology: TI- Nspire
Content:
Lines with gradients: Parallel and Perpendicular lines
Concept of function and its inverse – Domain, Range and graph
Inverse Functions
Sketching graphs and interpreting the key features of the graph.
Using technology to find: Point of Intersection, Zeros and sums and differences
Duration of the module: 4 Hours (Two sessions of 2 hours each)
Fee: SGD 160/-
Module Code: IBMAIM5
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the students learn to model real-life events using different functions. The students learn to model various functions: Linear, Quadratic, Cubic, Exponential growth and decay models, Direct and inverse variation and Sinusoidal Models. This module will help the students to determine the suitable models in varied contexts and it is helpful to do well in the three exam papers and IA.
Prior Learning: Students are expected to be well versed with forming an equation and solving for the variable and an idea of domain and range
Technology: TI- Nspire
Content:
Linear and Quadratic Models ( Inclusive of piece-wise functions)
Exponential Growth and Decay Models
Direct/ Inverse variations and Cubic Models
Sinusoidal Models
Applications: Population Growth, Boyle’s law, Spread of Virus
Duration of the module: 4 Hours (Two sessions of 2 hours each)
Fee: SGD 160/-
Module Code: IBMAIM6
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn to identify the model that fits the best to the applications. Students also learn to develop and fit the model, determine reasonable domain and reflect on the validity of models. This module helps to acquire the skills required to do well in paper 2, 3 and IA.
Prior Learning: Students are expected to be well versed with linear, quadratic, cubic and exponential models
Technology: TI- Nspire
Content:
Modelling process in TI and by hand
Developing and fitting the model
Testing and reflection of model
Duration of the module: 4 Hours (Two sessions of 2 hours each)
Fee: SGD160/-
Module Code: IBMAIM7
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn in depth on transforming the functions. Students engage to apply the transformation operations to composite functions. They also extend their learning from modelling to Natural logarithmic, Logistic, Sinusoidal and piece wise models. Interpretation of log-log and semi-log graphs. This module helps the student acquire the skills and concept to do well in the three exam papers.
Prior Learning: Students are expected to be well versed with the modelling techniques and functions concept to begin with this module.
Technology: TI Nspire calculator software
Content:
Composite functions and its inverse with domain restrictions
Transformations of Graphs: Translation, Reflection, Rotation and Composite transformations
Natural Logarithmic Models, Sinusoidal Models, Logistic and Piecewise models.
Linearizing data using logarithms – Determination if the exponential or a power relationship
Interpretation of Log-Log and Semi-log graphs
Duration of the module: 6 Hours ( 3 Sessions of 2 hours each)
Fee: SGD 240/-
Module Code: IBMAIM8
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn the concept of geometry and trigonometry: Properties of shapes,Volume and surface area, Non-right angled trigonometry, applications of Right and Non right angled trigonometry, Angles of elevations and Depression. This module helps the students to do well in the three exam papers.
Prior Learning: Students are expected to be well versed with pythagoras theorem,bearings and circle properties
Technology: TI- Nspire Calculator
Content:
Distance between two points in 3D and Size of an angle between two intersecting lines
Volume and surface area of 3D solids
Sine and Cosine rule, Area of Triangle
Applications of Non right angled trigonometry
Circle properties
Duration of the module: 4 Hours (Two sessions of 2 hours each)
Fee: SGD 160/-
Module Code: IBMAIM9
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn to understand the voronoi diagrams and its properties. Students learn to add an additional site to existing diagram, Nearest neighbour interpolation and its applications. This module helps the students understand the complete concept of voronoi and they will be able apply in the three exam papers.
Prior Learning: Students are expected to be well versed with the concept of Perpendicular bisectors.
Technology: Geogebra
Content:
Voronoi diagram – Sites, cells, vertices and edges.
Adding site to an existing voronoi diagram
Nearest neighbourhood interpolation
Applications to Voronoi Diagrams
Duration of the module: 4 Hours (Two sessions of 2 hours each)
Fee: SGD 160/-
Module Code: IBMAIM10
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn geometric transformations in composition. Students will be introduced to vectors and its properties. Vector applications in kinematics and in motion of 2 and 3 Dimensions. Calculation of scalar product and angle between two vectors is taught in depth. This module develops the confidence in the students to do well in the three exam papers.
Content:
Geometric transformations using matrices ( Including compositions)
Geometric Interpretation of the determinant
Introduction to vector and scalar
Types of vectors
Vector applications: Kinematics and constant velocity
Scalar and Vector Product
Duration of the module: 8 Hours (Four sessions of 2 hours each)
Fee: SGD 320/-
Module Code: IBMAIM11
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn about graph theory concepts in detail about the properties of graphing, tree and cycle algorithms, eulerian trails and application oriented contexts: Chinese postman problem and Travelling salesman problem. This module helps HL students to do well in the three exam papers and IA.
Content:
Graph theory : Graphs, Vertices, Adjacent edges and degree of vertex.
Simple, Complete, weighted, directed and in-out graphs
Subgraphs and Trees
Adjacency Matrices, Walks, Number of walks between two vertices
Weighted adjacency tables for undirected and directed graph
Tres and Cycle algorithms
Eluerian trails and circuits
Hamiltonian paths and cycles
Minimum Spanning Trees
Kruskal’s and Prim’s Algorithm
Chinese Postman Problem – Determination of shortest route
Travelling salesman Problem – Nearest neighbour algorithm
Deleted vertex algorithm
Duration of the module: 16 Hours (Eight sessions of 2 hours each)
Fee: SGD 640/-
Module Code: IBMAIM12
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn about the data handling and its properties. Student also gain confidence in the concepts of sampling methods and their effectiveness. They also learn to estimate the measure of central tendency and correlation, corresponding to the regression line. Statistics covers about 55% of the syllabus and with this module, students will gain an in-depth understanding to do well in the three exam papers.
Prior Learning: Students are expected to be well versed with the basic understanding of data and the scatter plot’s strength estimation.
Content:
Population and its types – Reliability and effectiveness
Interpretation of outliers and the effective uses sampling methods
Presenting Discrete and Continuous Data – Frequency Distribution Table.
Histograms- Cumulative frequency to find medians, percentiles and IQR
Production and understanding of Box and whisker plot
Measure of central tendency – Estimation from grouped data
Measures of Dispersion
Linear Correlation – Bivariate data
Pearson’s Product- moment correlation coefficient
Scatter Diagrams
Equation of Regression
Parameters of Regression
Duration of the module: 8 Hours (Four sessions of 2 hours each)
Fee: SGD 320/-
Module Code: IBMAIM13
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn about the probability and its characteristics. Students will be given a deeper insight of combined events and number of occurrences using venn diagrams and outcome tables. Students gain skills to be able to solve expected value for discrete data. Students who opt to do this develop confidence to do well in the three exam papers and the IA.
Prior Knowledge: Students are expected to know basic probability operations and construct venn diagrams with given data.
Content:
Trial, outcomes and types of events
Venn Diagrams, Tree Diagrams and Sample space
Combined Events and Mutually Exclusive events
Conditional Probability
Independent Events
Discrete Random Variables – Expected value – Applications
Duration of the module: 6 Hours (Three sessions of 2 hours each)
Fee: SGD 240/-
Module Code: IBMAIM14
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn and gain a deep understanding of distributions – Binomial and Normal and its curves. Students use technology to determine the properties and applications of the curves. Students also learn about inverse normal calculations as well. This module helps the students to do well in the three exam
papers and the IA.
Technology: TI nspire calculator
Content:
Binomial Distribution
Mean and variance of the Binomial Distribution
Normal Distribution and the curve
Properties of Normal Distribution
Normal Probability calculations
Inverse normal calculations
Duration of the module: 8 Hours ( Four sessions of 2 hours each)
Fee: SGD 320/-
Module Code: IBMAIM15
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn about spearman’s correlation coefficient and the appropriateness and limitation of pearson’s correlation coefficient and the effect of outliers on each. Students will be introduced to Null and Alternative hypotheses. This module help the students in gaining rich conceptual understanding of the testing methods in statistics that can be extended to IA and provides insights to do well in the three exam papers.
Prior Knowledge: Students are expected to be aware of the probability, correlation and outliers.
Content:
Spearman’s rank correlation coefficient
Appropriateness and Limitations of Pearson’s moment correlation coefficient.
Formulation of Null and Alternative Hypotheses
The Chi square test for independence and goodness of fit test
T-test
Use of p-value to compare populations
One tailed and two tailed tests
Duration of the module: 10 Hours (Five sessions of 2 hours each)
Fee: SGD 400/-
Module Code: IBMAIM16
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn about data collection methods and identifying the variables. Students will be able categorize the numerical data. They also learn reliability and validity tests and learn to perform regression with nonlinear functions using technology. Students will learn the importance of coefficient of determination. This module helps the students to do well in the three exam
papers and the IA.
Prior Learning: Students need to be well versed with sampling techniques and basic statistics of measures of dispersion.
Technology: TI nspire calculator
Content:
Design and validation – Surveys and Questionnaires
Data Analysis and identifying variables
Reliability and validity tests
Regression with nonlinear functions
Sum of square residuals as a measure of best fit
Coefficient of Determination
Evaluation of R2 using technology
Duration of the module: 8 Hours (Four sessions of 2 hours each)
Fee: SGD 320/-
Module Code: IBMAIM17
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn about transformations and combinations. Students learn linear combinations in n random variables, Variance of combinations for independent random variables. They also learn central limit theorem and confidence intervals for the mean of a normal population. This module helps the students to do well in the paper 2 and paper 3.
Prior Knowledge: Students are expected to be well versed with Random variables concept and mean, median and variance.
Content:
Linear transformation of a single random variable
Variance of linear combination of ‘n’ independent random variables
Unbiased estimates
Central Limit theorem
Confidence intervals of a mean of the population
Duration of the module: 8 Hours (Four sessions of 2 hours each)
Fee: SGD 320/-
Module Code: IBMAIM18
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn about poisson distribution – mean and variance. Students will be introduced to critical values and critical regions. Students also explore tests for population for binomial and poisson distribution. Students use technology to test the hypotheses and calculate the Type- I and II errors.
Prior Knowledge: Students should be well versed with distributions and curves
Content:
Poisson distribution – Mean and Variance
Sum of two Independent poisson distribution
Critical values and critical regions
Test for Proportion using Binomial Distribution
Test for population mean by using poisson distribution
Use of technology to test hypotheses
Type I and II errors including calculations of their probabilities.
Duration of the module: 10 Hours (five sessions of 2 hours each)
Fee: SGD 400/-
Module Code: IBMAIM19
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn about the transition of matrices and the application to markov chains by calculating the steady state and long term probabilities and long term probabilities by repeated multiplication of the transition matrix. This module helps the students to do well in the paper 2 and
paper 3.
Prior Knowledge: Students are expected to be well versed with operations of matrices
Technology: TI nspire calculator
Content:
Transition Matrices
Power of transition Matrices
Regular Markov Chains
Probability matrices
Steady state and long term probabilities.
Duration of the module: 4 Hours (Two sessions of 2 hours each)
Fee: SGD 160/-
Module Code: IBMAIM20
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student will be introduced to calculus and its properties in detail with limits, principles and properties. Students will get a very deeper insight on functions and change in the curve. This module helps the students to do well in the IA.
Prior Knowledge: Students need to be well versed with functions and the properties like gradients and tangents
Technology: TI nspire Calculator
Content:
Introduction to Limits concept
Properties of Limits
Estimation of limits from different graphs and tables
Introduction to the concept of rate of change
Duration of the module: 4 Hours (Two sessions of 2 hours each)
Fee: SGD 160/-
Module Code: IBMAIM21
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the students will be introduced to calculus and its properties in detail with differentiation and the increase and decrease of functions. Students will be exploring graphs and tables to estimate the rate of change in a function. Students will also learn about the Tangents and normals of the functions. This module helps the students to do well in the three exam
papers and the IA.
Prior Knowledge: Students need to be well versed with functions and the properties like gradients and tangents
Technology: TI nspire Calculator
Content:
Introduction to Differentiation
Interpretation of increasing and decreasing functions
Graphical Interpretation in rate of change
Introduction to derivative – Power function
Tangents and Normals
Duration of the module: 6 Hours (Three sessions of 2 hours each)
Fee: SGD 200/-
Module Code: IBMAIM22
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the students learn the concept of maxima and minima and perform optimisation in different contexts. This module helps the students to do well in the three exam
papers and the IA.
Prior Knowledge: Students need to be well versed with differentiation and its rules.
Technology: TI nspire Calculator
Content:
Values of ‘x’ where gradient of a curve is zero
Solutions for f’(x) = 0
Local Minimum and Maximum points
Optimisation in different contexts
Duration of the module: 6 Hours (Three sessions of 2 hours each)
Fee: SGD 240/-
Module Code: IBMAIM23
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student will be introduced to calculus- Integration and its properties in detail with rules and derivatives. Students get a very deeper insight on integrating functions. This module helps the students to do well in the three exam
papers and the IA.
Prior Knowledge: Students need to be well versed with differentiation and the rules
Technology: TI nspire Calculator
Content:
Introduction to Integration as Anti Differentiation
Anti differentiation with boundary conditions
Integration rules
Definite integrals using technology
Area of trapezoidal rule
Duration of the module: 6 Hours (Three sessions of 2 hours each)
Fee: SGD 240/-
Module Code: IBMAIM24
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn the differentiation in extension required for HL course. Students learn derivatives and second derivatives to distinguish between maximum and minimum points. Students also explore the definite and indefinite integration and area of region and volume of revolution. They also learn to solve kinematics problems. This module helps the students to do well in the three exam
papers and the IA.
Prior Knowledge: Students need to be well versed with differentiation-Integration and the rules.
Technology: TI nspire Calculator
Content:
Derivatives of sinx, cosx,tanx, ln x, ex, xn.
Chain rule, Product rule and Quotient Rule
Related rates of Change
Second derivative
Second derivative test to distinguish maximum and minimum point
Definite and Indefinite Integration of xn
Area of the region enclosed by the curve
Volume of revolution
Kinematics Problems
Duration of the module: 16 Hours (Eight sessions of 2 hours each)
Fee: SGD 640/-
Module Code: IBMAIM25
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student learn to set up the differential equations and solve them by separation of variables. Students also learn to construct slope fields and their diagrams in the given context. They also explore and apply Euler’s method to approximate first order differential equations and phase portraits. This module helps the students to do
well in the three exam papers and the IA.
Prior Knowledge: Students need to be well versed with Functions and Differentiation-Integration
Technology: TI nspire Calculator
Content:
Setting up a model/differential equation from a context
Solving by separation of variables
Slope fields
Euler’s method of Approximation
Phase Portraits
Sketching Trajectories using phase portraits
Solutions for second order differential equations
Duration of the module: 10 Hours (Five Sessions of 2 hours each)
Fee: SGD 400/-
Module Code: IBMAIM26
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student will be provided with a differentiated set of Paper 1 style model paper and skills needed to solve this paper will be observed and helped to develop the student’s potential to attempt this paper. Time management and organisational skills are focused to develop a comfortable approach to successfully do Paper 1.
Technology: TI nspire Calculator
Content:
Unit – Number & Algebra
Unit – Functions
Unit – Geometry & Mensuration
Unit – Statistics & Probability
Unit – calculus
Duration of the module: 10 Hours (Five sessions of 2 hours each)
Fee: SGD 400/-
Module Code: IBMAIM27
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student will be provided with a differentiated set of Paper 2 style model paper. They develop skills needed to solve this paper, whcih will be observed and they get help to develop potential to do this paper well. Time management, technology and organisational skills are focused to develop a comfortable approach to successfully do in Paper 2.
Technology: TI nspire Calculator
Content:
Unit – Number & Algebra
Unit – Functions
Unit – Geometry & Mensuration
Unit – Statistics & Probability
Unit – calculus
Duration of the module: 10 Hours (Five sessions of 2 hours each)
Fee: SGD 400/-
Module Code: IBMAIM28
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student will be provided with a differentiated set of Paper-3 style model paper and skills needed to solve this paper will be observed and helped to develop the student’s potential to attempt this paper. Time management, technology and organisational skills are focussed to develop a comfortable approach to successfully attempt the Paper-3.
Technology : TI nspire Calculator
Content:
Unit – Number & Algebra
Unit – Functions
Unit – Geometry & Mensuration
Unit – Statistics & Probability
Unit – calculus
Duration of the module: 10 Hours (Five sessions of 2 hours each)
Fee: SGD 400/-
Module Code: IBMAIM29
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
In this module, the student need to come up with a streamlined IA topic. This module helps the student to progress with the topic and involve all the criteria. Students will draft a short report based on a topic chosen and focuses on mathematical connections and concepts.
Technology: TI nspire Calculator
Duration of the module: 10 Hours (Five sessions of 2 hours each)
Fee: SGD 400/-
Module Code: IBMAIM30
Mode of Teaching: Online
How to enrol: Fill in the form and submit for slots and payment details CLICK HERE
